Switch the Knights
Your goal is to switch the positions of the three white knights with the positions of the three black knights. What is the least number of moves required to do this?

The least number of moves required to switch the positions of the knights is 16. An example is shown below.
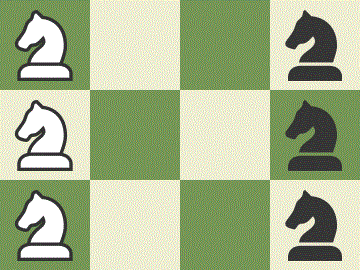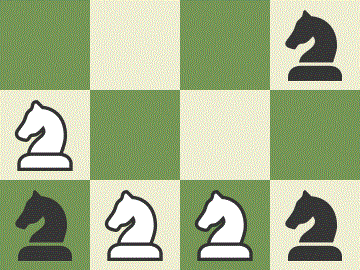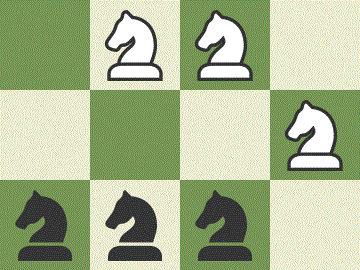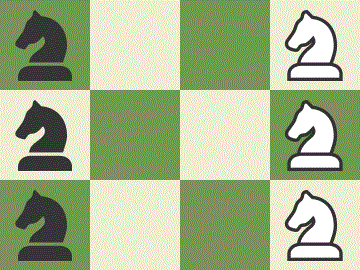
Next, we prove that it is impossible to switch the positions of the knights with less than 16 moves. Since the white knights occupy 2 black and 1 white squares, they need to end up on 2 white and 1 black squares, and each knight must make at least 2 moves in order to get to the opposite side, the total number of moves for the white knights should be an odd number, larger or equal to 2+2+2=6. The same applies to the total number of moves for the black knights. Therefore, the only possible way to get a total number of moves less than 16 is if both the white knights and the black knights move exactly 7 times.
We assume it is possible to switch the positions with 7+7=14 moves in total. Then, the white knight on A2 and one of the white knights on A1, A3 should make 2 moves each, and the third white knight should make 3 moves and land on a white square, either D1 or D3. Without loss of generality, we assume the knight on A1 makes 3 moves: A1-B3-C1-D3. Then, the knight on A2 should make 2 moves: A2-C3-D1, and the knight on A3 should make 2 moves: A3-B1-D2.
We make the same argument for the black knights. Since it is impossible that the white knight on A1 moves along the trajectory A1-B3-C1-D3 and also the black knight on D3 moves along the trajectory D3-C1-B3-A1, we conclude that the black knight on D1 should make 3 moves: D1-C3-B1-A3, the black knight on D2 should make 2 moves: D2-B3-A1, and the black knight on D3 should make 2 moves: D3-C1-A2.
This is only possible if:
- the knight on A1 moves to C1 after the knight on D3 moves to A2
- the knight on D3 moves to A2 after the knight on A2 moves to C3
- the knight on A2 moves to C3 after the knight on D1 moves to B1
- the knight on D1 moves to B1 after the knight on A3 moves to D2
- the knight on A3 moves to D2 after the knight on D2 moves to B3
- the knight on D2 moves to B3 after the knight on A1 moves to C1
We get a contradiction which means that the least number of moves is 16.
We do not know where this puzzle originated from. If you have any information, please let us know via email.


