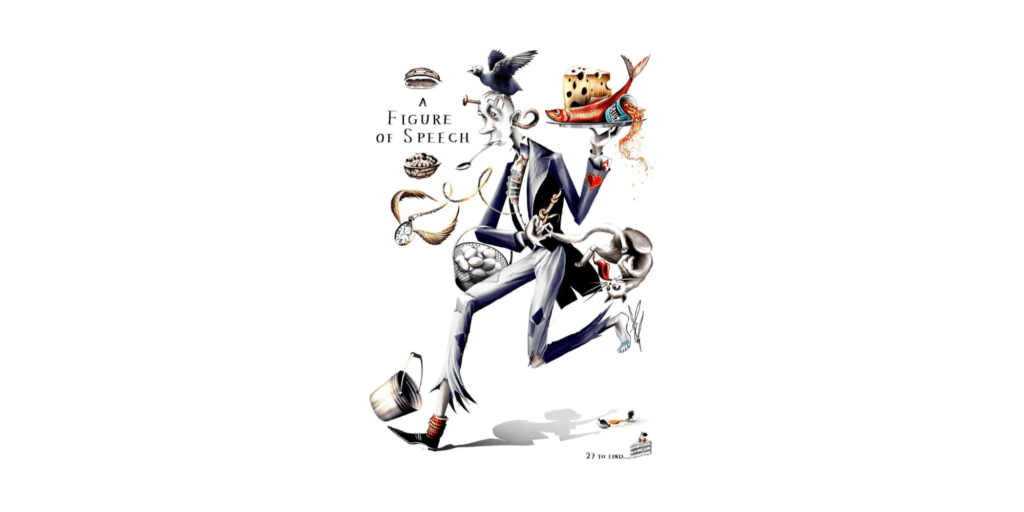The World’s Easiest Quiz
100 Guests in 99 Rooms
One hundred people entered a hotel that had 99 rooms, and each of them asked for their own room. In order to solve the problem, the bellboy did the following:
He asked the 100th guest to wait for a while with the 1st guest in room number 1, so that there were 2 guests inside. Then he took the 3rd guest to room number 2, the 4th guest to room number 3, and so on, until finally taking the 99th guest to room number 98. At the end he returned to room number 1 and took the 100th guest to room number 99, which was still vacant.
How could everybody get their own room?
SOLUTION
The two people who were taken to room number 1 were guest 1 and guest 100. Thus, the 2nd guest ended up without a room.
Beaten and Burned
Ripped from my mother’s womb,
Beaten and burned, I become a bloodthirsty killer.
What am I?
SOLUTION
The answer is IRON ORE.
Died on the Fourth of July
During a trivia night, the following question appeared:
“Among the first five US presidents, Washington, Adams, Jefferson, Madison, and Monroe, there were 3 who died on the fourth of July. Can you guess which ones?”
After nobody answered the question, the following hint was given:
“These three presidents were consecutive ones.”
Then, all contestants immediately managed to answer the question. Can you?
SOLUTION
Because of the way the question was worded, it was clear that the fifth president, James Monroe, was one of them. Otherwise, the trivia question would have been “among the first four US presidents” instead of “among the first five”. Since the three presidents that died on fourth of July were consecutive ones, they were Jefferson, Madison, and Monroe.
27 Figures of Speech
Find 27 figures of speech in the illustration by Ella Baron below.
SOLUTION
- A piece of cake
- An ace up one’s sleeve
- Big cheese
- Play one’s cards close to one’s chest
- Cats got your tongue
- Cherry on the cake
- Crow over
- Cut the chase
- Don’t put all your eggs in one basket
- Hit the nail on the head
- Have (one’s) plate full
- Heart on one’s sleeve
- Holding a cat by the tail
- In a nutshell
- Kick the bucket
- Make ends meet
- Picture is worth a thousand words
- Joker in the pack
- Put a bug in someone’s ear
- Put one’s best foot forward
- Red herring
- Silver spoon in your mouth
- Spill the beans
- Tie in knots
- The shoe is on the other foot
- Time flies
- You can’t make an omelette without breaking egg
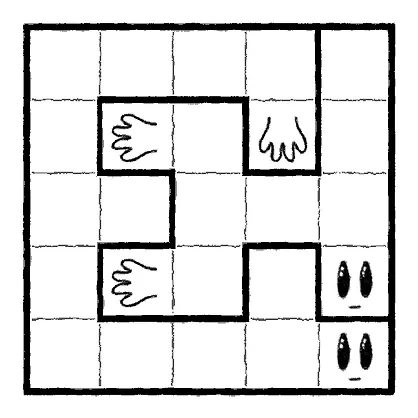
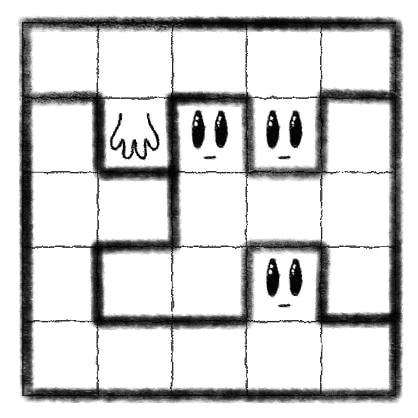
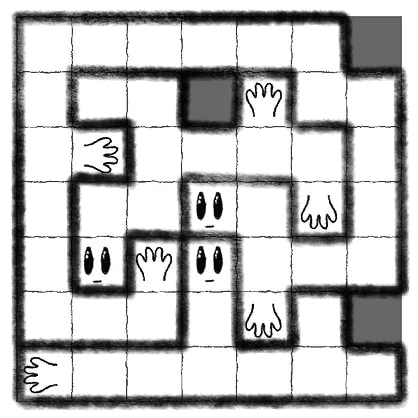
Limbs
Partition the grid into disjoint “creatures”, according to the following rules:
- Each creature is defined as a shape of 4 connected branches that are each 1 cell wide.
- For each creature, one of the branches ends up with a HEAD (always clued) and the other three branches end up with LIMBS (whenever clued, their directions matter).
- A creature can never occupy a 2×2 region of cells and can never touch itself.
Examine the first example, then solve the other three puzzles.
SOLUTION
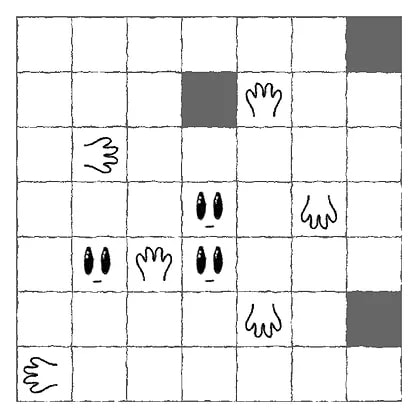
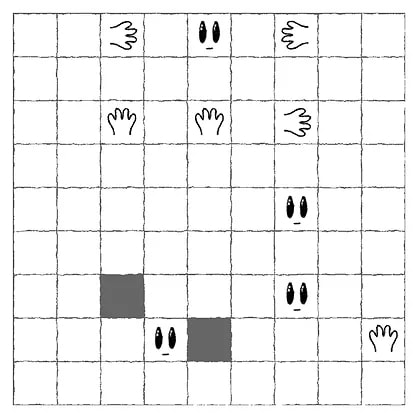
The solutions are shown below.
Do Not Add E
Add a single letter at the end to create a meaningful word. You CANNOT add the letter E.
S E Q U E N C _
SOLUTION
You should add the letter F above the underscore to create the letter E and get the word SEQEUNCE.
1 2 3 4 5 ?
Can you guess what the question mark should be replaced with? The answer is not 6.
| 1 | 3 | 5 |
| 2 | 4 | ? |
SOLUTION
The missing symbol is R, as in a car’s gears.
Hidden Objects Birds
Can you find the bird with the party hat?
SOLUTION
The solution is shown below.
Mammals in Cages
You walk into a room where there are three mammals held in cages:
- a trained dog doing tricks
- a monkey playing with blocks
- a lab rat traversing a maze
Which is the most intelligent mammal in the room?
SOLUTION
The answer is… YOU.