Puzzle Archives - Puzzle Prime

Out of Time

Section: Brain Teasers Category: Chess

Difficulty: Advanced

In the position below, Black played a move, but right before he pressed the clock, he ran out of time. However, the judge declared a draw instead of awarding a victory to the opponent. Why?

SOLUTION

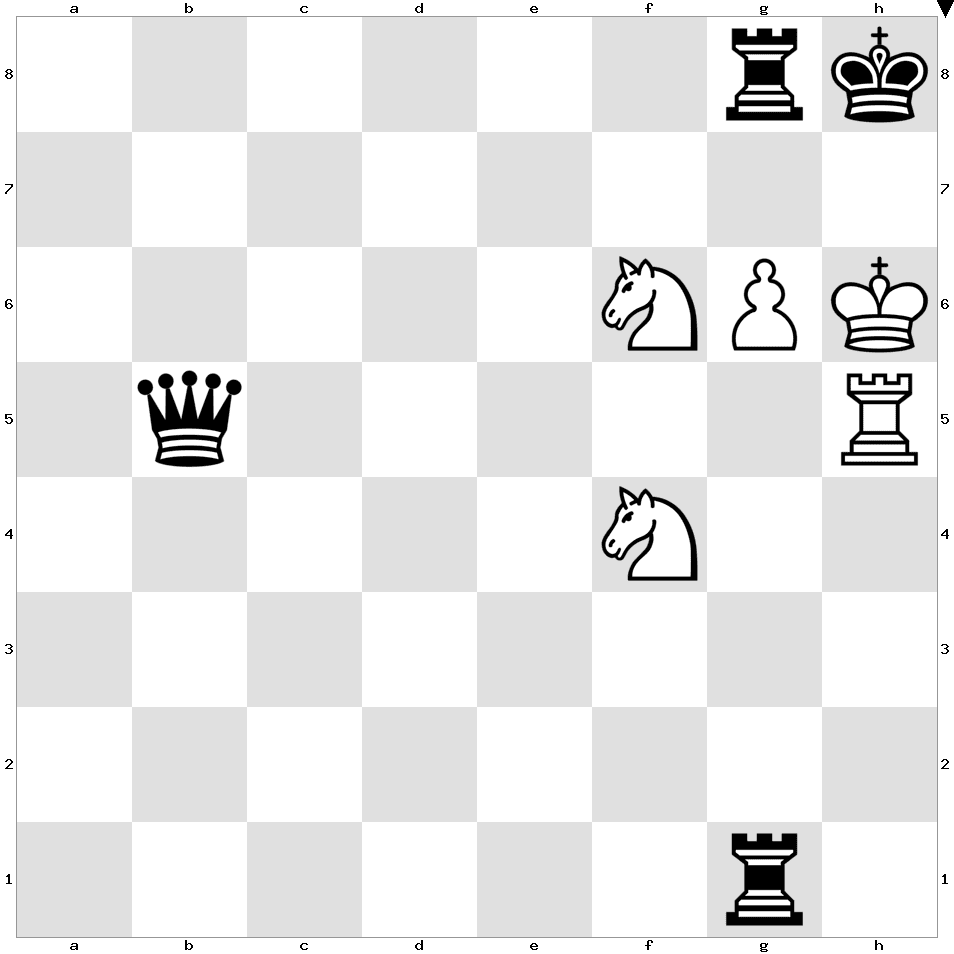
The rules of FIDE state that if a player runs out of time, their opponent wins the game IF they have a path to victory. If there is no sufficient material, e.g. a King and a Knight against a King, then the game is declared a draw.

In this position, Black played Rxg6 which forces the moves:

… Rxg6+
Nxg6+ Rxg6+
Kxg6+ Qxg6+
Kxg6

This leaves White with a King and a Knight against Black’s King. Thus, White did not have a path to victory and the game was declared a draw.

Puzzle Prime October 28, 2023

0 Comments

Unconscious and Bleeding

Section: Brain Teasers Category: Lateral

Difficulty: Medium

A man is found unconscious in front of a store at two in the morning. His head is bleeding and there is a brick laying next to him. When the police arrive, they carry the man to jail. Why did they arrest him?

SOLUTION

The man was a burglar who tried to break the store’s glass with the brick. The glass turned out to be bullet proof, so the brick bounced back and hit him in the head, knocking him out.

Unknown Author October 25, 2023

1 Comment

Dr. Riesen’s Rebuses 2

Section: Casual Puzzles Category: Rebus

Difficulty: Medium

Can you figure out what common phrases these rebuses represent?

SOLUTION

The answers are:

A drop in the bucket
First edition
Kick the bucket
Feeling under the weather
Don’t cut corners
Pillow talk
Don’t cry over spilled milk
Jump the gun
Spill the beans
A piece of cake
Back to square one
Hold your horses
Catch my drift
Let the cat out of the bag
Face the music
Barking at the wrong tree

Guillaume Riesen October 22, 2023

0 Comments

Pretty Words

Section: Brain Teasers Category: Insight

Difficulty: Medium Tags: Words

Can you recognize the four words below?

ZO-ZO
ZOOZ
OON
ZCZ

SOLUTION

If you rotate the screen by 90°, you will read vertically:

ONION
NOON
ZOO
NUN

Unknown Author October 19, 2023

5 Comments

Seven Loaves of Bread

Section: Brain Teasers Category: Mathematics

Difficulty: Easy

There are 7 loaves of bread that need to be shared equally among 12 people. How would you do this if you are not allowed to split any loaf into 12 pieces?

SOLUTION

Split 4 of the loaves into 3 pieces and the other 3 loaves into 4 pieces. Then, give each person one of both types of pieces.

Unknown Author October 16, 2023

0 Comments

Everything and Nothing

Section: Casual Puzzles Category: Riddle

Difficulty: Advanced

Turn me on my side and I am everything. Cut me in half and I am nothing. What am I?

SOLUTION

The answer is THE DIGIT 8.

If you turn it on its side, you will get the symbol infinity. If you cut it in half horizontally, you will get the number 0.

Unknown Author October 13, 2023

1 Comment

Death Cult

Section: Brain Teasers Category: Mathematics

Difficulty: Hard

A thousand people stand in a circle in order from 1 to 1000. Number 1 has a sword. He kills the next person (Number 2) and gives the sword to the next living person (Number 3). All people keep doing the same until only one person remains. Which number survives?

SOLUTION

First, we note that if the number of people is a power of 2, then the first person will survive every round. The greatest power of 2 that is less than 1000 is 512. Therefore, after 488 people die, there will be 512 remaining and the first one to kill the 489-th person will survive. This person has number 1+2×488=977.

Unknown Author October 10, 2023

25 Comments

A String Around a Rod

Section: Brain Teasers Category: Mathematics

Difficulty: Medium Tags: Geometry

A string is wound around a circular rod with circumference 10 cm and length 30 cm. If the string goes around the rod exactly 4 times, what is its length?

SOLUTION

Imagine the circular rod is actually a paper roll and the string is embedded inside the paper. When we unroll it, we get a paper rectangle 30cm×40cm with the string embedded along the diagonal. Using the Pythagorean theorem, we find that the length of the string is 50cm.

Unknown Author October 7, 2023

0 Comments

A Heart That Doesn’t Beat

Section: Casual Puzzles Category: Riddle

Difficulty: Medium

I have a heart that doesn’t beat.
I have a mouth that doesn’t speak.
I have a head that doesn’t think.

What am I?

SOLUTION

The answer is ARTICHOKE.

Unknown Author October 4, 2023

0 Comments

FEATURED

Imprisoned Logicians

Section: Brain Teasers Category: Deduction

Difficulty: Expert Tags: Featured

Two friends, logicians – Ein and Stein – get imprisoned in two distant cells in a castle. Both cells have just one door, and a window with 8 bars in the first cell, and 12 bars in the second cell. The first day both logicians get the same letter from the prison master:

“The total number of bars in the two prison cells in this castle is either 18 or 20. Starting tomorrow, every morning I will go first to Ein and then to Stein, and will ask how many bars the other logician has. If one of you answers correctly, I will immediately let both of you leave the castle. If one of you answers incorrectly, I will execute both of you. Of course, you can always decide not to answer and just stay imprisoned.
I have sent a copy of this letter to you and your friend. There is no point in trying to communicate with him – your cells are far away from each other, and he won’t hear you.”

Will the logicians manage to escape the castle eventually? When will they do it?

SOLUTION

Solution coming soon.

Unknown Author October 1, 2023

8 Comments