Resting Tire
Which tire does not move when the car turns right?
SOLUTION
The answer is THE SPARE TIRE.
Category: Insight
We do not know where this puzzle originated from. If you have any information, please let us know via email.
Shuffling Cards
52 cards – 2 of clubs to Ace of clubs, 2 of diamonds to Ace of diamonds, 2 of hearts to Ace of hearts, and 2 of spades to Ace of spades – are arranged in a deck. We shuffle them in the following manner:
- We take the top card and put in a random place inside the deck.
- Once we get to the King of spades and put it somewhere in the deck, we stop.
Show that this method shuffles the deck uniformly, i.e. every permutation has the same chance to appear.
SOLUTION
Notice that at all times the cards below the King of spades are shuffled uniformly. Therefore at the end, after we put the King of spades in a random place inside the deck, the entire shuffle will be uniform as well.
Cover the Grid
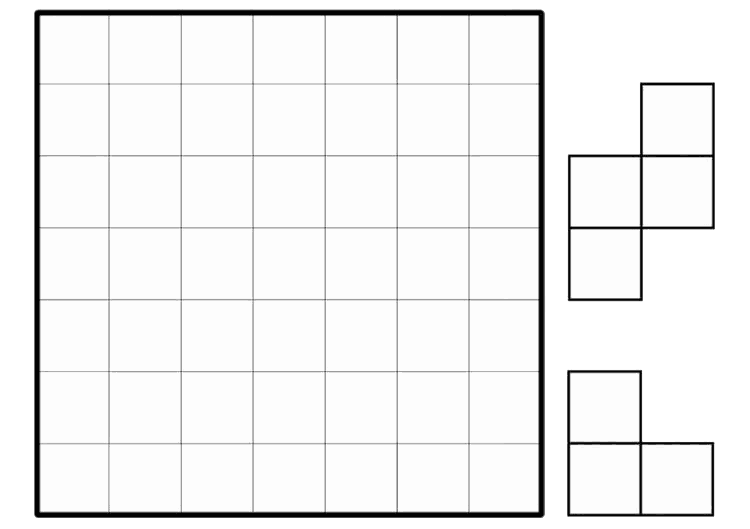
You must cover a 7×7 grid with L-shaped triminos and S-shaped tetrominos, without overlapping (flipping and rotating is permitted). What is the minimum number of pieces you can use in order to do this?
Remark: All pieces must be placed entirely on the board.
SOLUTION
Consider all cells in the grid which lie in an odd row and odd column – there are 16 of them. Since each of the two pieces can cover at most 1 of these cells, we need at least 16 pieces. Giving an example with 16 pieces is easy.
10 Prisoners, 10 Keys, 2 Weeks
One day, the warden of a prison is, like most wardens in puzzles, feeling a little capricious and decides that he wants to get rid of his prisoners, one way or another. He gathers all the prisoners in the yard and explains to them – “Tonight, I will go to each of you, hand you a key, and tell you who has your key. Each day after that, while the others are out of the cells and no one is watching, I will allow each of you to place your key in someone else’s cell – and each night, you may collect the keys in your own cell. If at any point, you are certain that everyone has the key to their own cell, you may summon me, at which point each of you will open your own cell and walk free. If anyone has the wrong key, everyone will be executed then and there. You may discuss your strategy before tonight, but afterward
That night, just as promised, the warden went to each cell and gave each prisoner a key. As he handed each prisoner the key, he whispered to them the name of the person possessing the key to their cell. The keys were entirely indistinguishable from one another, but that was okay, because the prisoners had not counted on being able to tell anything about them. Indeed, the prisoners all seemed confident.
What was their strategy? How could they beat the warden’s game?
SOLUTION
We assume the cells in the prison are arranged in a circle. The prisoners agree every day each of them to pass the key they receive to their left neighbor, except for their own key which they keep. It is easy to figure out which key is their own since they can easily calculate when they will receive it. For example, if prisoner 8 knows that his key is at prisoner 3 in the beginning, then he will get it on the 5th day. Therefore, within 10 days, all prisoners will have their own keys.
Source:
High Tide
A boat has a ladder with six rungs on it. The rungs are spaced one foot from each other, the lowest one starting a foot above the water. The tide rises with 10 inches every 15 minutes.
How many rungs will be still above the water 2 hours later?
SOLUTION
Six rings – the ship and the ladder will be rising with the tide.
Seven Dwarfs
In one house deep in the forest
SOLUTION
The seventh dwarf is also playing chess; 2 people are needed for this.
Birds on a Tree
There are 10 birds on a tree. One hunter shoots at them and hits 2. How many birds are left on the tree?
SOLUTION
Zero – the remaining 8 birds get scared and fly away.
Traveling Salesmen
Between every pair of major cities in Russia, there is a fixed travel cost for going from either city to the other. Traveling salesman Alexei Frugal starts in Moscow and visits all cities exactly once, choosing every time the cheaper flight to a city he has not visited so far. Salesman Boris Lavish starts in St Petersburg and visits all cities exactly once, choosing every time the most expensive flight to a city he has not visited so far. Can Alexei end up spending more money than Boris after they end their journeys?
SOLUTION
No, it is impossible. For every trip of Alexei we will choose a trip of Boris, which costs at least as much.
Let the number of cities is n and Alexei visits them in order 1 -> 2 -> … -> n.
If Boris visits city n-1 before city n, then pair Alexei’s trip (n-1, n) with Boris’ trip (n-1, #). Notice that C(n-1, n) < C(n-1, #).
If Boris also visits city n-2 before city n, then pair Alexei’s trip (n-2, n-1) with Boris’ trip (n-2, #). Notice that C(n-2, n-1) < C(n-2, n) < C(n-2, #).
Continue like this until get to a city k which Boris visits after city n. Then pair Alexei’s trip (k, k+1) with Boris’ trip (n, #). Notice that C(k, k+1) < C(k, n) < C(n, #).
Next, check whether Boris visits city k – 1 before city k, and pair Alexei’s trip (k-1, k) with either (k-1, #) or (k, #). Continue like this, until pair all of Alexei trips with more expensive Boris trips.
The Poisoned Glass
You are given 4 identical glasses, completely filled with transparent, odorless liquids. Three of the liquids are pure water, and the fourth is poison, which is slightly heavier. If the water glasses weigh 250 grams each, and the poisoned glass weighs 260 grams, how can you figure out which one is which, using a measuring scale just once?
SOLUTION
Empty the first glass, fill around 1/4th of it with liquid from the second glass, and the rest 3/4ths with liquid from the third glass. Then, measure the first and fourth glasses simultaneously. If their total weight is:
– 500 grams -> the first glass is (was) the poisoned one
– between 500 and 505 grams -> the second glass is the poisoned one
– between 505 and 510 grams -> the third glass is the poisoned one
– 510 grams -> the fourth glass is the poisoned one
Source:
