Fish in a Pond
There are 5 fish in a pond. What is the probability that you can split the pond into 2 halves using a diameter, so that all fish end up in one half?

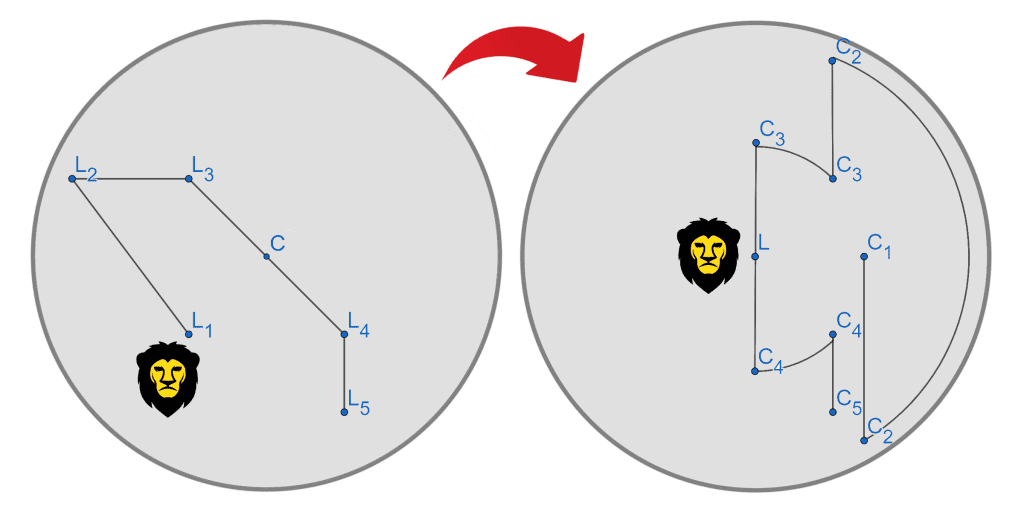
Let us generalize the problem to N fish in a pond. We can assume that all fish are on the boundary of the pond, which is a circle, and we need to find the probability that all of them are contained within a semi-circle.
For every fish Fᵢ, consider the semi-circle Cᵢ whose left end-point is at Fᵢ. The probability that all fish belong to Cᵢ is equal to 1/2ᴺ⁻¹. Since it is impossible to have 2 fish Fᵢ and Fⱼ, such that the semi-sircles Cᵢ and Cⱼ contain all fish, we see that the probability that all fish belong to Cᵢ for some i is equal to N/2ᴺ⁻¹.
When N = 5, we get that the answer is 5/16.