Pass Through a Card
Take a small piece of paper – 4in by 6in, and scissors. Can you cut the paper in such a way, so that you can pass through it
SOLUTION
Yes, you can, as shown on the image below.
Category: Puzzles
Harry Houdini (1874 – 1926) was a Hungarian-born American illusionist and stunt performer, noted for his sensational escape acts. He first attracted notice in vaudeville in the US and then as "Harry Handcuff Houdini" on a tour of Europe, where he challenged police forces to keep him locked up. Soon he extended his repertoire to include chains, ropes slung from skyscrapers, straitjackets under water, and having to escape from and hold his breath inside a sealed milk can with water in it.
Be brave!
Which is the best move for White in this position?
SOLUTION
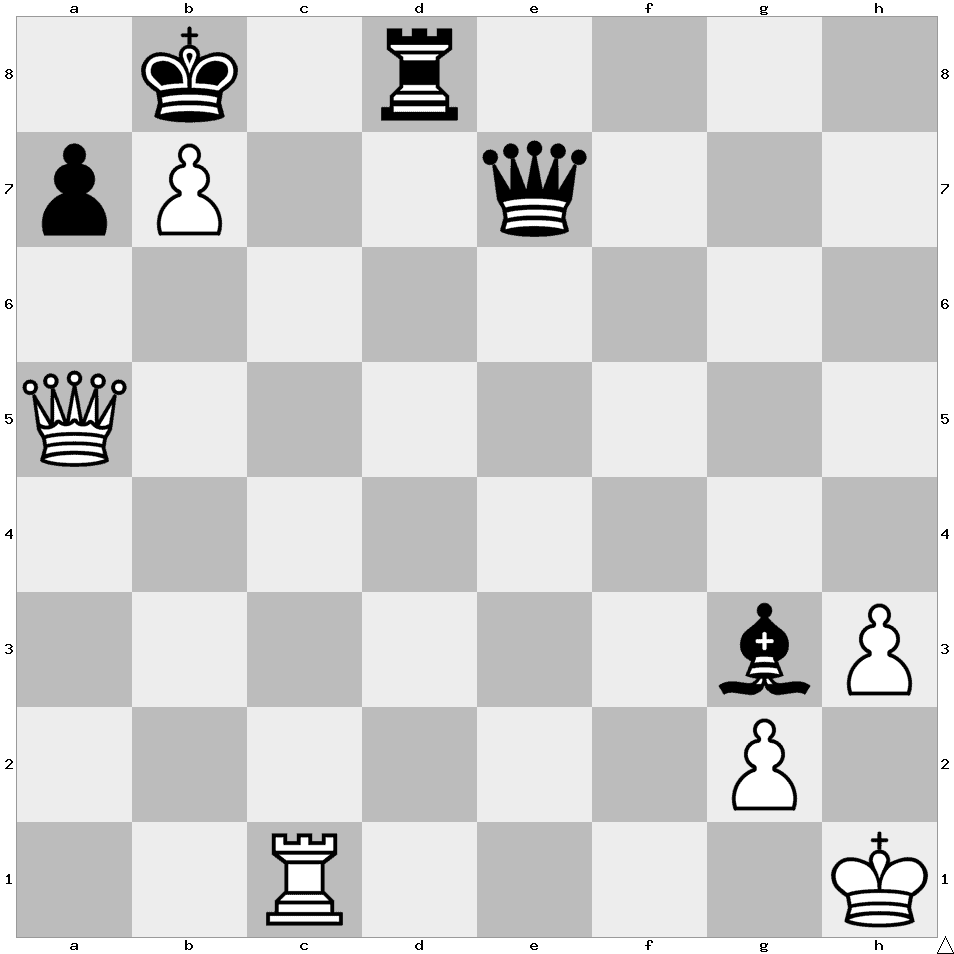
The best move is 1. Rc8+. Then either 1. … Kxb7 2. Rxd8, or 1. … Rxc8 2. Qxa7 Kxa7 3. bxc8 = N+, and Black loses both the Rook and the Queen.
Hidden Objects Piles 2
Can you find the objects which are hidden in the images below?
SOLUTION
The solution is shown below.
Complex Timepiece
A sundial has the fewest moving parts of any timepiece. Which has the most?
SOLUTION
The hourglass, it has thousands of grains of sand.
The Pasta Puzzle
You have 10 strings of pasta left on your plate. You randomly start tying up their ends, until there are no loose ends anymore. What is the average number of loops which are created?
SOLUTION
The expected (average) number of loops at the end of the procedure is equal to the expected number of loops created after the first tying, plus the expected number of loops created after the second tying, etc. After each tying, the number of non-loop strings decreases by 1, and then the probabilities to create a new loop are 1/19, 1/17, 1/15, etc. Therefore, the answer is the sum 1/19 + 1/17 + 1/15 + … + 1/3 + 1/1 ~ 2.1.
Versatile Word
What four-letter word can be written forward, backward or upside down, and can still be read from left to right?
SOLUTION
The word NOON.
Green House
There was a green house. Inside the green house there was a white house. Inside the white house there was a red house. Inside the red house there were lots of babies. What is it?
SOLUTION
The answer is WATERMELON.
Brick Measurement
Imagine you have three identical bricks and want to find the common length of their main diagonals. What is the easiest way to do this using just a measuring tape?
SOLUTION
PLace the bricks as shown on the image below, then measure the marked segment.
