Slicing a Bagel
Get a bagel and a knife, then cut the bagel in two pieces which end up linked to each other.
SOLUTION
The solution is presented HERE.
Category: Puzzles
George William Hart (1955) is an American geometer who expresses himself both artistically and academically. He is also an interdepartmental research professor at the State University of New York in Stony Brook, New York. His artistic work includes sculpture, computer images, toys (e.g. Zome) and puzzles. His sculptures have been featured in articles in The New York Times, Science News, and other publications around the world.
Non-Transitive Dice
This is a non-transitive dice set, i.e. every dice in it is weaker than some other dice. Can you design a non-transitive set with only 3 dice?
Remark: “Weaker” means that it loses more often than it wins.
SOLUTION
The simplest solution is given by:
2, 2, 4, 4, 9, 9;
1, 1, 6, 6, 8, 8;
3, 3, 5, 5, 7, 7.
Another solution is given by the so-called “Miwin’s dice”. They are as follows:
1, 1, 3, 5, 5, 6;
2, 3, 3, 4, 4, 5;
1, 2, 2, 4, 6, 6.
Puzzle Sum 22
What state does this sum spell?
SOLUTION
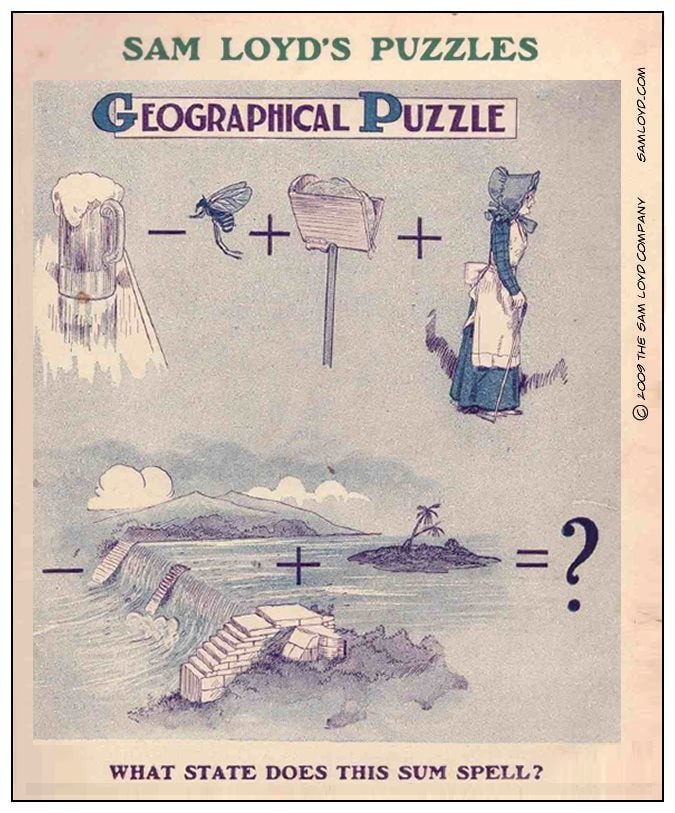
BEER – BEE + HOD + DAME – DAM + ISLAND = RHODE ISLAND
Socks in the Drawer
You have a drawer with 10 pairs of black socks and 10 pairs of white socks. How many times do you need to blindly reach inside the drawer and take out a sock, so that you get a matching pair?
SOLUTION
Only 3 times. Once you have two socks of the same color, they already form a matching pair.
Two Sisters
Two sisters we are, one is dark and one is fair,
In twin towers dwelling we’re quite the pair,
One from land and one from sea,
Tell us truly, who are we?
SOLUTION
The answer is SALT AND PEPPER.
Hidden Objects Crowds
Can you find the objects hidden in the three pictures below? You are looking for:
- 1 panda among the heavy metal artists
- 1 panda among the storm troopers
- the Queen among her Corgies
SOLUTION
Coming soon.
0 > 2, 2 > 5, 5 > 0
0 > 2, 2 > 5, 5 > 0. What is this?
SOLUTION
This is the game “Rock, Paper, Scissors”. Rock (0 fingers) beats scissors (2 fingers). Scissors (2 fingers) beats paper (5 fingers). Paper (5 fingers) beats rock (0 fingers).
Cow and Rope
A cow is tied to a 4 meter long rope. There is food, 20 meters away from the cow. However, the cow manages to go to the food and eat it. How come?
SOLUTION
The rope is not tied to anything but the cow, so the cow is free to go to the food.
The Missing Digit
The number 229 has 9 digits, all different. Which digit is missing?
Bonus: Is the number 9991 prime?
SOLUTION
Let the missing digit be m. Every number and the sum of its digits give the same remainder when divided by 9. The number 229 = 32 * 644 gives remainder 5 when divided by 9, and therefore 9 divides (0 + 1 + 2 + … + 9) – 5 – m = 40 – m. Thus, the missing digit is 4.
Bonus: 9991 = 10000 – 9 = 1002 – 32 = (100 – 3)(100 + 3) = 97 * 103. Therefore the number 9991 is not prime.
Cover the Table
100 coins are placed on a rectangular table, such that no more coins can be added without overlapping. Show that you can cover the entire table with 400 coins (overlapping allowed).
SOLUTION
Since we can not place any more coins on the table, each point of it is at distance at most 2r from the center of some coin, where r is the radius of the coin. Now shrink the entire table twice in width and length, then replace every shrunk coin with a full sized one. This way the small table will be completely covered because every point of it will be at distance at most r from the center of some coin. Add three more of these smaller tables, covered with coins, to create a covering of the big table.