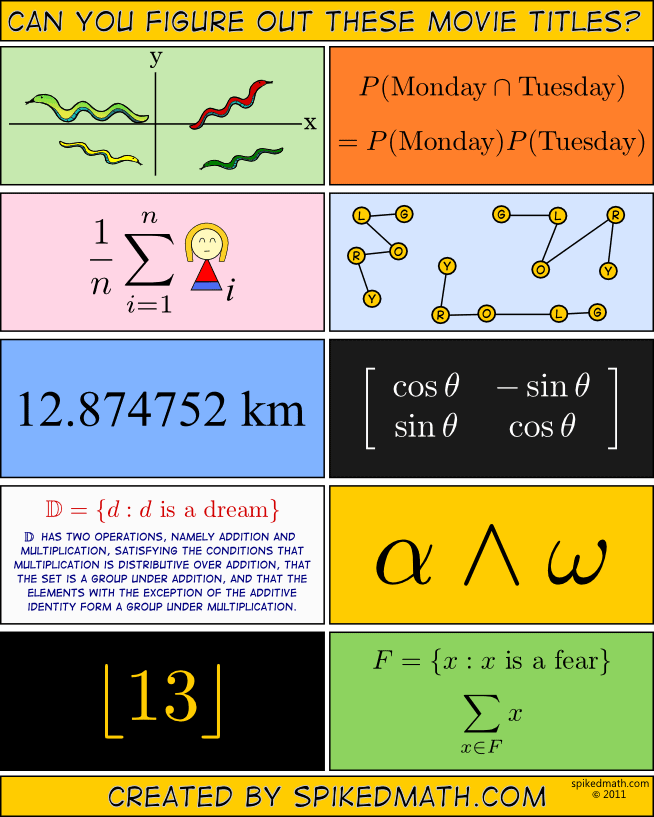
Spiked Math Comics is a math comic dedicated to humoring, educating, and entertaining geeks.
Can you recognize which famous movies are depicted by these images?
Suppose you are on a one-way circular racetrack. There are 100 gas cans randomly placed on different locations of the track and the total amount of gas in the cans is enough for your car to complete an entire circle. Assume that your car has no gas initially, but you can place it at any location on the track, then pick up the gas cans along the way and use them to fill the tank. Show that you can always choose a starting position so that you can complete an entire circle.
Imagine you put your car at any location, but instead with an empty tank, you start with enough gas to complete the circle. Then, simply track the amount of gas you have and locate the point on the track where it is the lowest. If you choose that location as a starting point, you will be able to complete the track.
You have 12 balls, 11 of which have the same weight. The remaining one is defective and either heavier or lighter than the rest. You can use a balance scale to compare weights in order to find which is the defective ball and whether it is heavier or lighter. How many measurement do you need so that will be surely able to do it?
It is easy to see that if we have more than 9 balls, we need at least 3 measurements. We will prove that 3 measurements are enough for 12 balls.
We place 4 balls on each side of the scale. Let balls 1, 2, 3, 4 be on the right side, and balls 5, 6, 7, 8 on the left side.
CASE 1. The scale does not tip to any side. For the second measurement we place on the left side balls 1, 2, 3, 9 and on the right side balls 4, 5, 10, 11.
If the scale again does not tip to any side, then the defective ball is number 12 and we can check whether it is heavier or lighter with our last measurement.
If the scale tips to the left side, then either the defective ball is number 9 and is heavier, or it is number 10/11 and is lighter. We measure up balls 10 and 11 against each other and if one of them is lighter than the other, then it is the defective one. If they have the same weight, then ball 9 is the defective one.
If the scale tips to the right side, the procedure is similar.
CASE 2. Let the scale tip to the left side during the first measurement. This means that either one of the balls 1, 2, 3, 4 is defective and it is heavier, or one of the balls 5, 6, 7, 8 is defective and it is lighter. Clearly, balls 9, 10, 11, 12 are all genuine. Next we place balls 1, 2, 5, 6 on one side and balls 3, 7, 9, 10 on the other side.
If the scale tips to the left, then either one of the balls 1, 2 is defective and it is heavier, or ball 8 is defective and lighter. We just measure up balls 1 and 2 against each other and find out which among the three is the defective one.
If the scale tips to the right, the procedure is similar.
If the scale does not tip to any side, then either the defective ball is 4 and it is heavier, or the defective ball is 8 and it is lighter. We just measure up balls 1 and 4 against each other and easily find the defective ball.
A teacher enters the classroom and sees on the first row two students sitting next to each other, looking completely identical. She asks them if they are twins, but the students simultaneously reply that they are not. After checking in the records, the teacher furthermore discovers that the two children have the same mother and father. What is the explanation?
The students are three of a triplet.
Darma and Greg are twins, Greg being the older one by just few moments. However, several times in their lives, Darma celebrated her Birthday two days before Greg did. How is this possible?
Darma and Greg were being delivered on a plane/ship which was passing the 180th meridian. This way Greg got born on 1st of March and Darma got born on 28th of February. Therefore, during leap years, Darma will celebrate her Birthday 2 days before Greg.
Can you arrange 6 new identical pencils in space such that every two of them touch each other? What if you have 7 pencils?
Remark: The construction doesn’t need to be stable.
Below you can see solutions for 6 and 7 pencils. The construction with 6 pencils is stable, the construction with 7 pencils – not.
You have unlimited number of knights, bishops, rooks and kings. What is the biggest number of pieces (any combination) you can place on a chessboard, so that no piece is attacked by another one?
If we put 32 knights on all black squares, then no two pieces will attack each other. Now let’s see that if we have more than 32 pieces, then there will be two which attack each other. Split the chessboard in 8 rectangular sectors of size 2×4. It is not hard to see that if we have more than 4 pieces in the same 2×4 sector, then 2 of them will attack each other. Therefore we can place at most 4 × 8 = 32 pieces on the chessboard.
A man stands in the center of a circular field which is encompassed by a narrow ring of water. In the water there is a shark which is swimming four times as fast as the man is running. Can the man escape the field and get past the water to safety?
Yes, he can. Let the radius of the field is R and its center I. First the man should start running along a circle with center I and radius slightly less than R/4. His angular speed will be larger than the angular speed of the shark, so he can keep running until gets opposite to it with respect to I. Then, the man should dash away (in a straight line) towards the water. Since he will need to cover approximately 3R/4 distance and the shark will have to cover approximately 3.14R distance, the man will have enough time to escape.
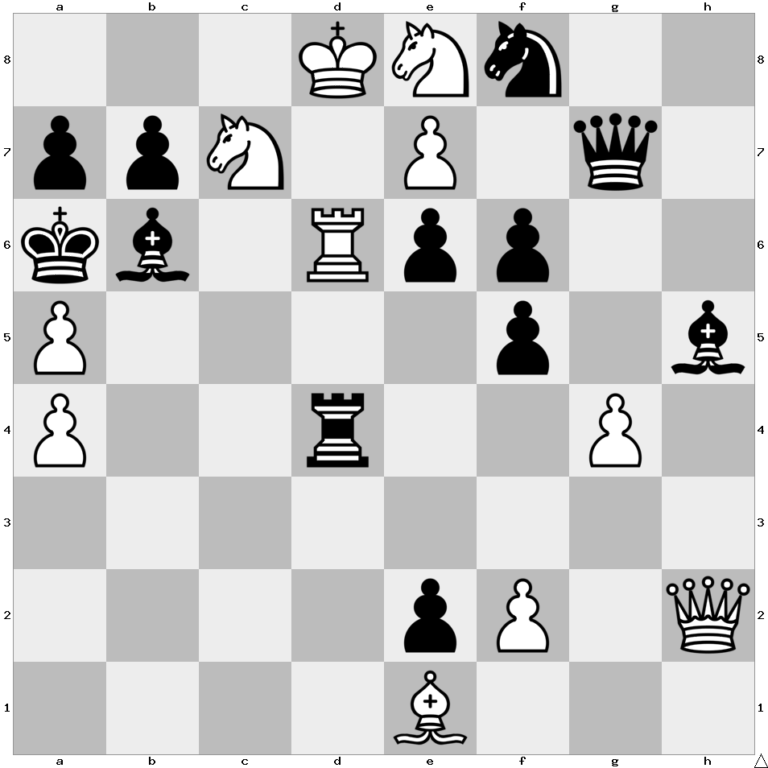
The following game is played under very specific rules – no pinned piece checks the opposite king. How can White mate Black in 2 moves?
First, White plays f3 and threatens mate with Qxe2. Indeed, blocking with the black rook on d4 will not help, because it will become pinned, which means that the rook on d6 will become unpinned, which will make the bishop on b6 pinned, and that will unpin the knight on c7, resulting in a mate. Below are listed all variations of the game.
1. … Rd5 2. Qxe2#
2. … Bxa5 2. Kc8#
3. … Bxc7 2. Nxc7#
4. … Bxe8 2. Kxe8#
5. … Qxe7+ 2. Kxe7#
6. … Rd2 2. Bxd2#
7. … Rxd6+ 2. Qxd6#
On the ground there is a stick and 10 ants standing on top of it. All ants have the same constant speed and each of them can travel along the entire stick in exactly 1 minute (if it is left alone). The ants start moving simultaneously straightforward, either towards the left or the right end of the stick. When two ants collide with each other, they both turn around and continue moving in the opposite directions. How much time at most would it take until all ants fall off the stick?
Imagine the ants are just dots moving along the stick. Now it looks looks like all dots keep moving in their initially chosen directions and just occasionally pass by each other. Therefore it will take no more than a minute until they fall off the stick. If any of them starts at one end of the stick and moves towards the other end, then the time it will take for it to fall off will be exactly 1 minute.
Please confirm you want to block this member.
You will no longer be able to:
Please allow a few minutes for this process to complete.
