We do not know where this puzzle originated from. If you have any information, please let us know via email.
Use exactly two threes (3) and two eights (8) to get the number 24. You can use multiplication (×), division (÷), addition (+), subtraction (-) signs, and brackets. You can not use any advanced arithmetic operations, such as exponential, factorial, etc.
The answer is shown below.
8 ÷ (3 – 8 ÷ 3) = 24
Two friend mathematicians meet each after
– I have 3 daughters, the product of their ages is 36.
– I can’t figure out how old they are, can you tell me more?
– Sure, the sum of their ages is equal to the number of my house.
– I know your house number, but still can’t figure out the ages of your daughters.
– Also, my eldest daughter is called Monica.
– OK, now I know how old your daughters are.
What ages are the three daughters of the mathematician?
Using the first clue, we find that there are 8 possibilities:
(1, 1, 36) -> sum 38
(1, 2, 18) -> sum 21
(1, 3, 12) -> sum 16
(1, 4, 9) -> sum 14
(1, 6, 6) -> sum 13
(2, 2, 9) -> sum 13
(2, 3, 6) -> sum 11
(3, 3, 4) -> sum 10
Since the second mathematician couldn’t guess the ages even after the second clue, the sum has to be 13. Therefore the only possible options are (1, 6, 6) and (2, 2, 9). However, the third clue suggests that there is an “eldest” daughter and then the correct answer is 2, 2 and 9.
100 prisoners are given the following challenge: They will be taken to a room and will be arranged in a column, such that each of them faces the backs of the prisoners in front. After that, black and red hats will be placed on their heads, and the prisoners will be asked one at a time what is the color of their hat, starting from the one at the back of the column. If a prisoner guesses his color correctly, he is spared; if not – he is executed. If every prisoner can see only the hats of the prisoners in front of him in the line, what strategy should they come up with, so that their losses are minimized?
There is a strategy which ensures that 99 prisoners are spared and there is 50% chance that one of them is executed. Clearly, one can not do better.
The strategy is as follows: The first prisoner (at the back of the line) counts the number of black hats worn by the prisoners in front. If the number is odd, he says “BLACK”. If the number is even, he says “RED”. Then, the second prisoner counts the black hats in front of him, figures out the color of his own hat, and answers the question.The third prisoner sees the number of black hats in front of him and uses this information, along with what the second prisoner’s hat is, to determine the color of his own hat. The prisoners continue in the same manner until all 99 prisoners in the front guess their hat colors correctly. The chance for survival of the first prisoner is 50%.
Manifold is a brilliant puzzle invented by Jerome Morin-Drouin. Based on the origami principle, the goal of Manifold is to fold the printed paper several times, so that eventually you will end up with a 4 × 4 square which is white on one side and black on the other. The Manifolds here are provided by The Incredible Company and are part of their Manifold game which contains a total of 100 puzzles. Click the images, download them, print them, and solve the puzzles.
Prepare a piece of paper with dimensions 2×4, then fold it four times to form 8 squares. Write on the squares in the top row the numbers 1, 8, 7, 4, and write on the squares in the bottom row the numbers 2, 3, 6, 5.
Now your task is to fold the piece of paper several times, so that the squares end up on top of each other, with the numbers appearing in ascending order top to bottom, and 1 face up.
Once you do this, try again with numbers 1, 8, 2, 7 on the top row, and 4, 5, 3, 6 on the bottom row.
Coming soon.
A chess king starts on one cell of a chessboard and takes a non-intersecting tour, passing through each square once, and ending up on the initial square. Show that the king has made no more than 36 diagonal moves.
The king must visit the 28 perimeter squares in order; otherwise, he will create a portion of the board which is inaccessible for him. However, he can not travel from one square to a neighboring one using only diagonal moves. Therefore, he must make at least 28 horizontal/vertical moves and at most 64 – 28 = 36 diagonal moves.
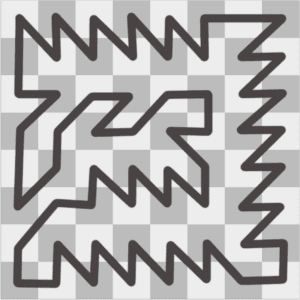
Can you draw uncountable many non-intersecting “8” shapes in the plane (they can be contained in one another)?
No, you can’t. For each “8” shape you can choose a pair of points with rational coordinates – one in its top loop and one in its bottom loop. Since no two “8” shapes can have the same corresponding pair of rational points, their number should be countable.
In a parliament
For each split of the people into 2 groups, compute the animosity level of each person by subtracting the number of enemies in the opposite group from the number of enemies in his own group. Then, split the people into 2 groups so that the total animosity level of all of them is as little as possible. If there is a person, who has more enemies in his own group than the opposite one, then by transferring him to the other group, we will reduce the total animosity level of the people and will get a contradiction.
An evil warden holds you as a prisoner but offers you a chance to escape. There are 3 doors A, B, and C. Two of the doors lead to freedom and the third door leads to lifetime imprisonment, but you do not which door is what type. You are allowed to point to a door and ask the warden a single yes-no question. If you point to a door that leads to freedom, the warden does answer your question truthfully. But if you point to the door that leads to imprisonment, the warden answers your question randomly, saying either “YES” or “NO” by chance. Can you figure out a way to escape the prison?
You can point towards door A and ask whether door B leads to freedom. If the warden says “YES”, then you open door B. It can not lead to imprisonment because this would mean that door A leads to freedom and the warden must have told you the truth. If the warden says “NO”, then you open door C. This is because either the warden lied, and then the imprisonment door is A, or he told you the truth, and then the imprisonment door is B.
There is an island on a planet and infinitely many planes on it. You need to make one of these planes fly all around the world and land back to the island. However, each of the planes can carry fuel which is enough to travel just half of the way, and fuel
3 planes are enough, label them A, B, C. They leave the island simultaneously in a clockwise direction, and after 1/8 of the way, A refuels B and C completely, then turns back towards the island. B and C continue to fly until they get to 1/4 of the way, where B refuels C completely and turns back towards the island. When C gets mid-way, A and B leave the island counter-clockwise, and after 1/8 of the way, A refuels B completely and turns back towards the island. B continues towards C, and when the two planes meet, they share their fuel, then fly together towards the island. In the meantime A arrives on the island, refuels completely, and starts flying again counter-clockwise towards B and C, so that it can meet them and give them enough fuel, so that all of them arrive safely on the island.
It is easy to see that 2 planes are not enough.
Please confirm you want to block this member.
You will no longer be able to:
Please note: This action will also remove this member from your connections and send a report to the site admin. Please allow a few minutes for this process to complete.
